In Memory computing with Memristive devices
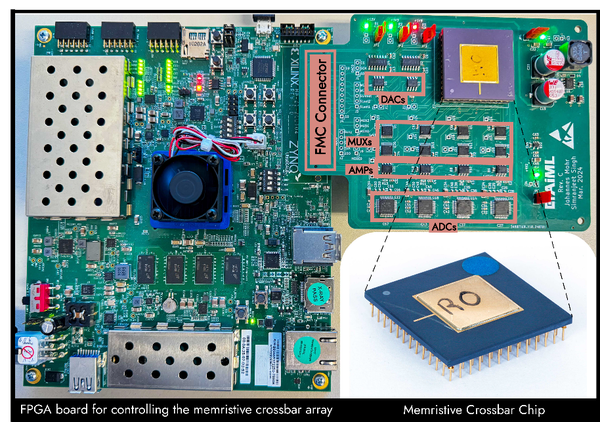
In-memory computing (iMC) using memristive devices represents a paradigm shift in computing architecture by merging storage and processing within the same physical location. These nanoscale resistive memory elements, typically based on HfO₂ or Ta₂O₅, perform computations through resistance modulation, enabling stateful logic operations such as OR, NOT, XOR, and NOT-IMPLY directly within memory arrays [1]. By eliminating the need for data transfer between discrete memory and processing units, this approach offers significant improvements in energy efficiency for data-intensive workloads. Furthermore, the inherent variability of resistive random-access memory (RRAM) is leveraged to improve the resilience of digital and analog machine learning models [2] and to develop RRAM based secure hardware primitives [3].
Our current research focuses on overcoming material variability challenges while advancing multi-bit operation, and hybrid CMOS-memristor designs to push the technology toward commercial viability in next-generation AI hardware and edge computing systems.
References:
[1] A. Bende et al., "Experimental Validation of Memristor-Aided Logic Using 1T1R TaOx RRAM Crossbar Array, "37th International Conference on VLSI Design and 2024 23rd International Conference on Embedded Systems (VLSID), Kolkata, India, 2024, pp. 565-570, doi: 10.1109/VLSID60093.2024.00100.
[2] T. Glint, G. Paul, A. Bende, R. Dittmann and V. Rana, "Resilience of Digital and Analog RRAM-Based ML Models to Device Variability: A Comparative Study," 2024 31st IEEE International Conference on Electronics, Circuits and Systems (ICECS), Nancy, France, 2024, pp. 1-4, doi: 10.1109/ICECS61496.2024.10848635.
[3] S. Singh et al., "Integrated Architecture for Neural Networks and Security Primitives using RRAM Crossbar," 2023 21st IEEE Interregional NEWCAS Conference (NEWCAS), Edinburgh, United Kingdom, 2023, pp. 1-5, doi: 10.1109/NEWCAS57931.2023.10198126.
