Hydrodynamik Simulation
In this two-dimensional simulation, three colloids with hard-sphere interaction are embedded in a solvent. The fluid is modelled by multi-particle collision dynamics (MPC), a particle-based mesoscopic hydrodynamics simulation technique. In this case, there are about 11,000 fluid particles, which are not shown. The fluid velocity field is represented by arrows. No-slip boundary conditions are employed for the fluid-colloid interaction. Since MPC naturally contains thermal fluctuations, the colloids are subject to Brownian motion.

Interactive simulation not yet available
Click on a colloid and drag it with your mouse through the fluid.
An external force proportional to the distance between the colloid and the current mouse position is applied (indicated by the red arrow).
When a colloid is dragged towards another colloid, a flow field emerges and momentum is transferred by the fluid to the second colloid that starts to move well before the hard colloid-colloid interaction becomes active. Due to the inherent many-body character of hydrodynamic interactions, the resulting forces acting on the other colloids depend on the relative positions of all colloids. Note that periodic boundary conditions are used, and the reference frame is co-moving with the center of mass of the whole system.
Mesoscale Simulations of Complex Fluids with Viscoelastic Solvent
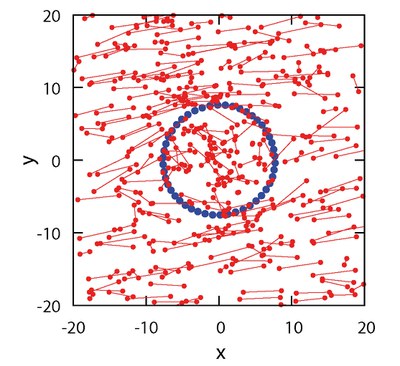
Under non-equilibrium conditions, soft matter systems exhibit both viscous and elastic characteristics -- they are viscoelastic. Prominent examples are polymer solutions and melts. For a colloidal suspension in a viscoelastic solvent, e.g., proteins in a cell, or colloids dispersed in polymer solutions, the situation is even more complex -- the solvent itself is now a complex fluid. To unravel dynamical and rheological properties of such complex systems requires a simplified mesoscopic model of a viscoelastic solvent.
We have extended the multiparticle collision dynamics (MPC) technique to shear-thinning viscoelastic solvents. Here, the normal MPC particles are replaced by dumbbells with harmonic or finite-extensible nonlinear elastic (FENE) springs. We have studied the properties of FENE-dumbbell fluids under simple shear flow. Shear-thinning behavior is found for shear rates larger than the inverse characteristic dumbbell relaxation time. We have applied this approach to the flow behavior of a colloid in a shear-thinning viscoelastic fluid.
Importance of Angular Momentum Conservation in Mesoscale Hydrodynamics Simulations
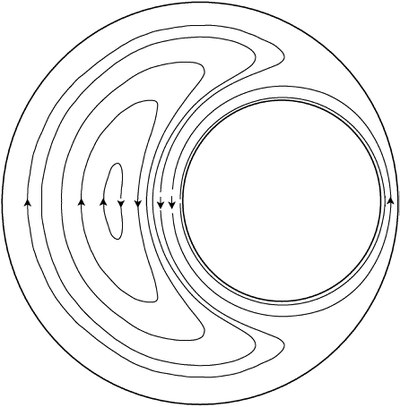
Angular momentum is conserved in fluids -- with a few exceptions such as ferrofluids in an external field. However, it can be violated locally in fluid simulations to reduce computational costs. We have developed a version of multiparticle collision dynamics (MPC), a particle- based simulation method, in which angular-momentum conservation can be switched on or off. To investigate the effects of angular-momentum violation, we have studied circular Couette flows between concentric and eccentric cylinders. Non-physical torques due to the lack of the angular-momentum conservation are found, whereas the velocity field is not affected. In addition, in simulations of fluids with different viscosities in contact, incorrect angular velocities are shown to occur. These results quantitatively agree with the theoretical predictions based on the macroscopic stress tensor, which contains an antisymmetric contribution in the absence of angular momentum conservation.
Multiparticle collision dynamics (MPC)

The dynamics of complex fluids is often governed by the hydrodynamic behavior of the solvent. Due to a large separation of length and time scales between the atomic scale of the solvent molecules and the mesoscopic scale of the solute, direct simulation approaches with atomistic solvent are prohibitively costly in computer time. This has stimulated the development of several mesoscale simulation techniques in recent years.
MPC is a particle-based mesoscale simulation technique. The fluid is modeled by N point particles. Positions and velocities evolve in discrete increments of time. The algorithm consists of two steps. In the streaming step the particles move ballistically. In the collision step, the particles are sorted into collision boxes, and the velocities relative to the centre of mass motion perform a random rotation.
