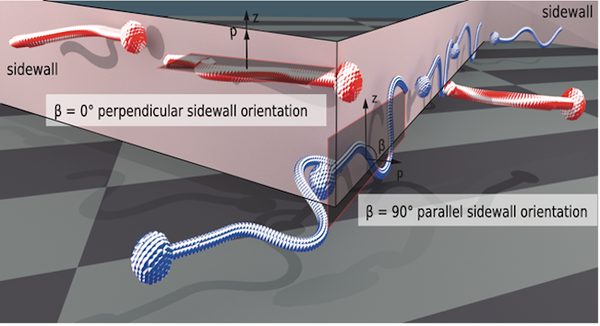
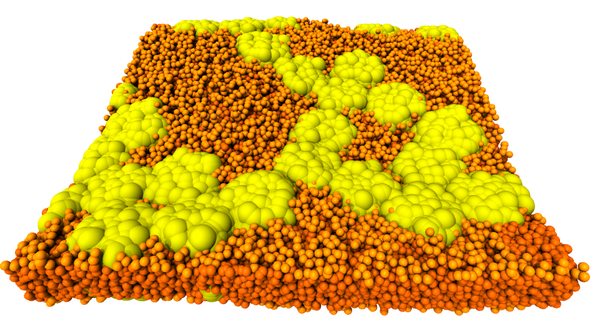
Membranen
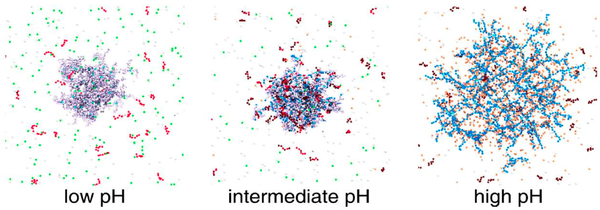
Eine grosse Vielfalt synthetischer und biologischer Nanoteilchen wechselwirkt mit der Zell- membran. Dies betrifft etwa die Invasion der Zelle durch Viren, oder die Nanotoxizität industriel- ler Nanoteilchen. Die Aufnahme in die Zelle wird durch viele Faktoren, wie Grösse, Form, und Oberflächen-Chemie, bestimmt.