Tissue Growth
Growing Materials

While other forms of growing materials exist (like bacterial colonies or possibly polymers during polymerization) the prime example of what we have in mind are biological tissues. Biological tissues form functional parts of organisms, composed of cells. They develop during embryogenesis and (most) are under constant renewal over the course of their lifetime. In the past decades it became more and more clear that physics, and especially mechanics, plays an important role in cellular and tissue growth. The group focuses on particle-based mesoscopic simulations of growing tissues. Cells are represented by point particles that repel themselves in order to grow. When a size threshold is reached, the cell divides. Otherwise cells behave like sticky soft colloids.This form of mechanical feedback on growth is sufficient to yield well-behaved mechanics-dependent growth.
Further reading:
Dissipative particle dynamics simulations for biological tissues: rheology and competition
Negative Homeostatic Pressure
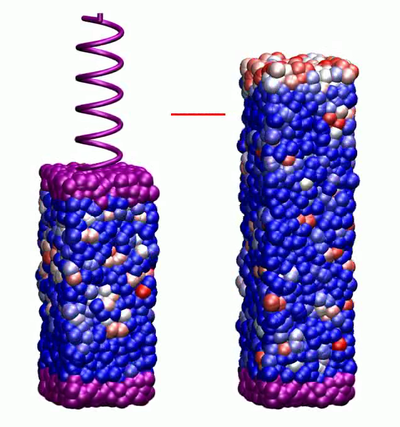
The homeostatic pressure is a key property in understanding the mechanics of tissues. In essence, it is the pressure a tissue self-developes when growing in a finite compartment. Or, in other words, it is the pressure at which apoptosis (cell death) and division balance. Using our particle-based simulations we found that this pressure can be negative, i.e. a homeostatic tension. Matching the simulations to experimental data reveals that this indeed the case for the tested cell lines, and that the observed pressure dependent growth rates seem to follow a relatively similar curve. The concept of negative homeostatic pressure is best understood from the neighbouring visualization of the simulations.
Further reading:
Tissue homeostasis: A tensile state
Mechanics of Competition

If two tissues with different mechanical propoerties grow in the same compartment, thei enter competition for space. The determening factor in the competition is the homeostatic pressure - the pressure to which the tissue grows when grwon in a finite compartement.
All other parameters the same, the tissue with the higher homeostatic pressure wins the competition. The simulations suggest, that from the interface profile between the two tissues, mechanical properties of the tissue can be deduced.
Further reading:
Interface dynamics of competing tissues
